Circle Geometry Formulas and Notes

Arc Angle

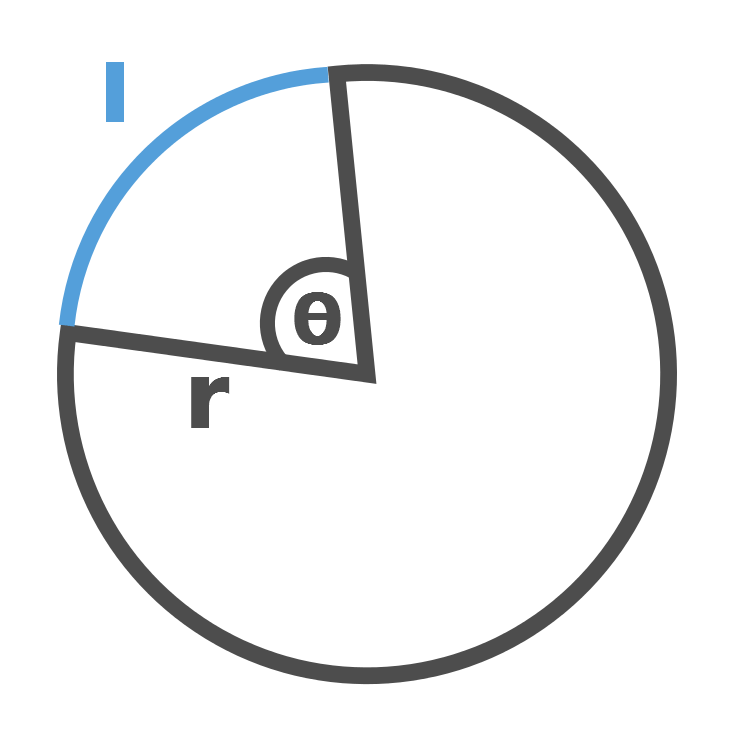
\displaystyle \theta = \frac{360 \degree l}{2 \pi r}

Arc Length

Arc Length(l) \displaystyle = \frac{ \theta \degree 2 \pi r}{360 \degree }

Sector Area

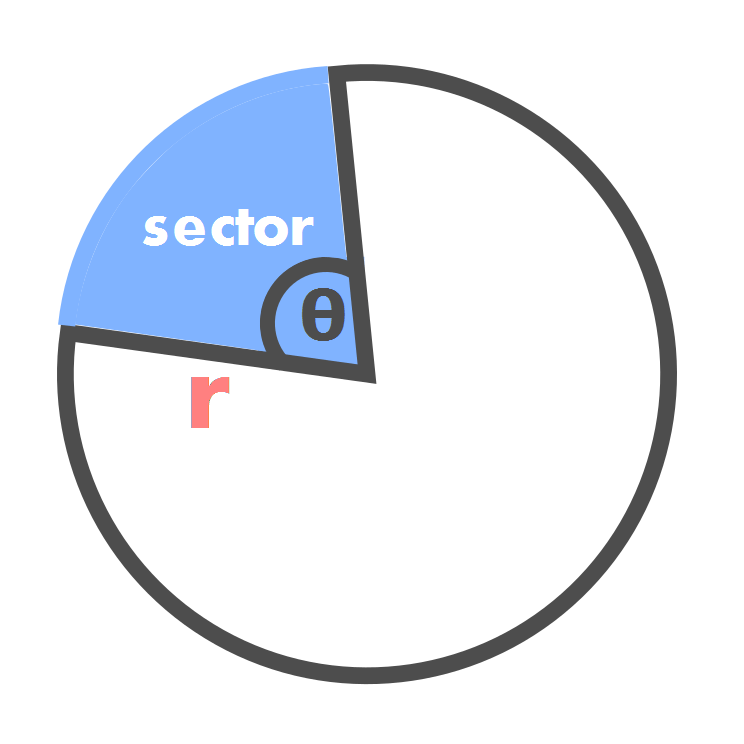
Sector Area \displaystyle = \frac{ \theta \degree \pi r^{2} }{360 \degree }

Segment

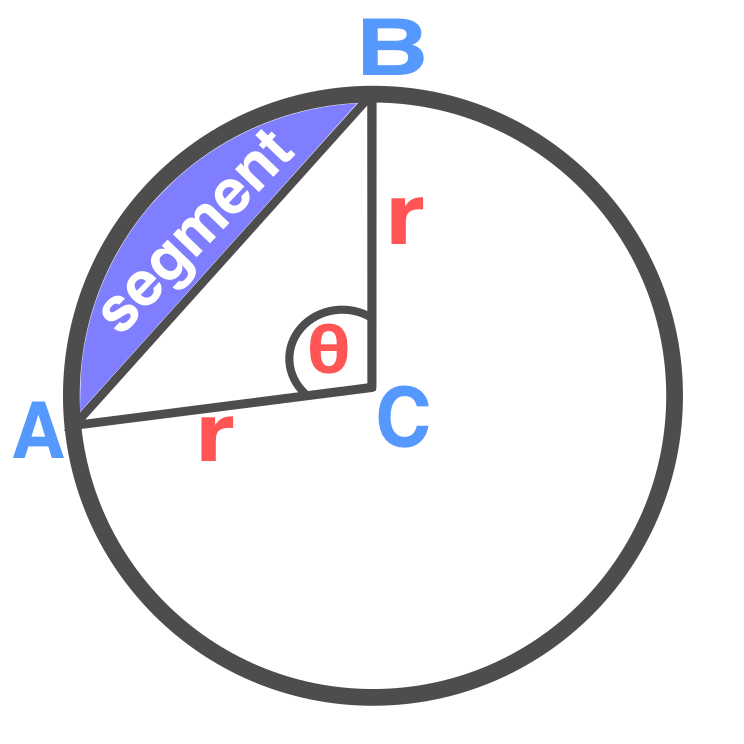
Segment = Sector - Area_ΔABC

Chord Length

Chord Length(AB) \displaystyle = \sqrt{(AC)^{2}+(BC)^{2}-2ab\cos( \theta)}

Inscribed Angle

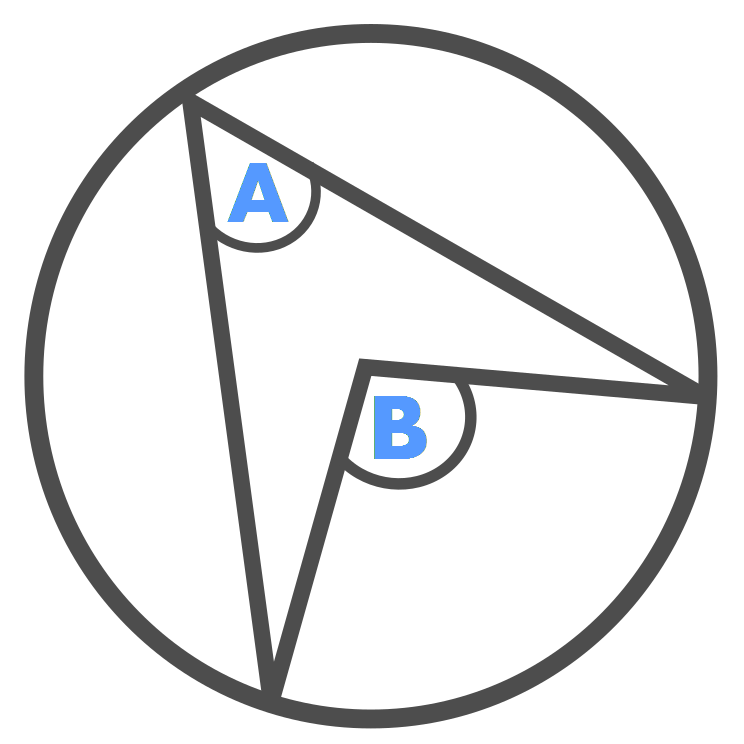
An inscribed angle is an angle with its vertex on the circle formed by two intersecting chords

Angle A \displaystyle = \frac{1}{2} Angle B

Tangent Chord Angle

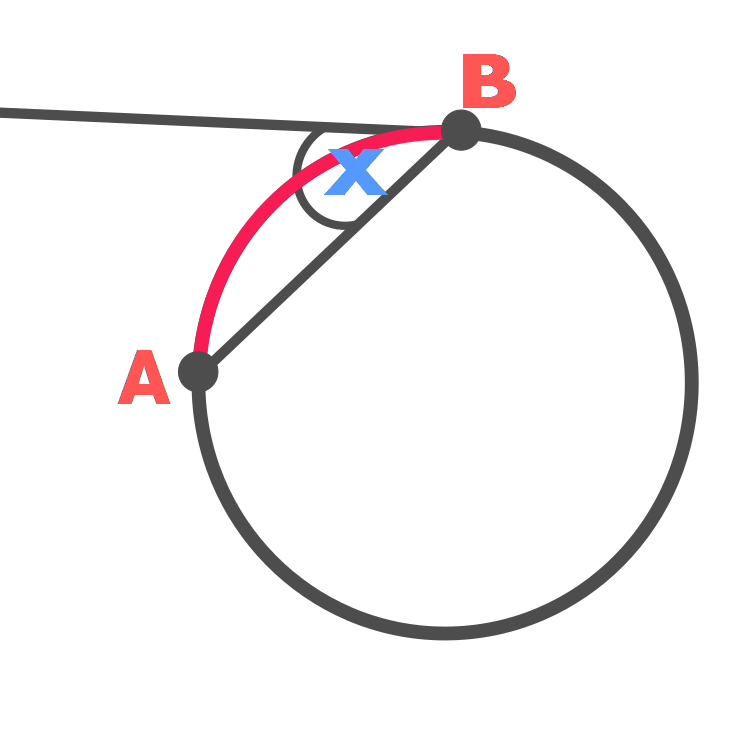
x = \frac{1}{2} (AB)

Intersecting Chords

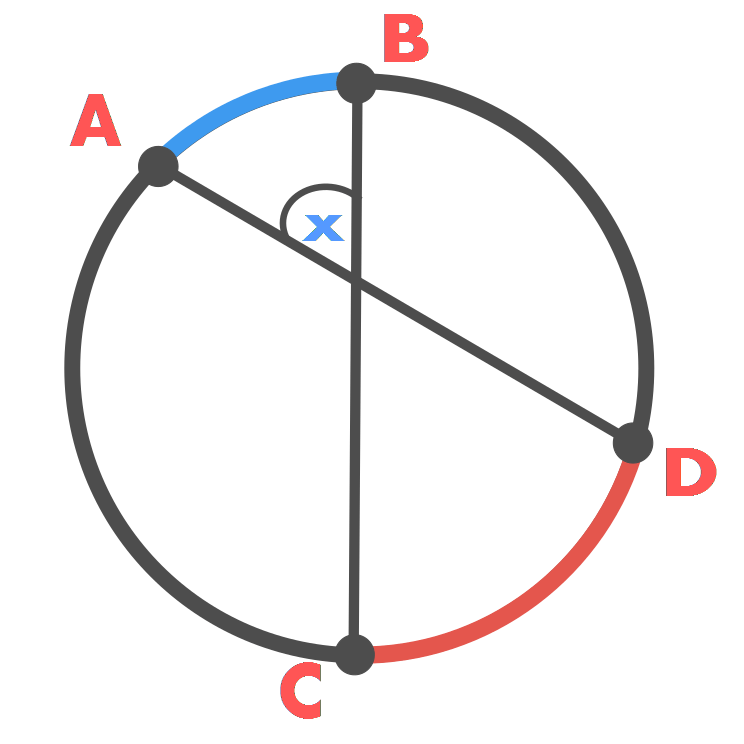
x = \frac{1}{2} (AB+CD)

Two Tangents

\angle ABC = \frac{1}{2} (ADC-AC)

Two Secants

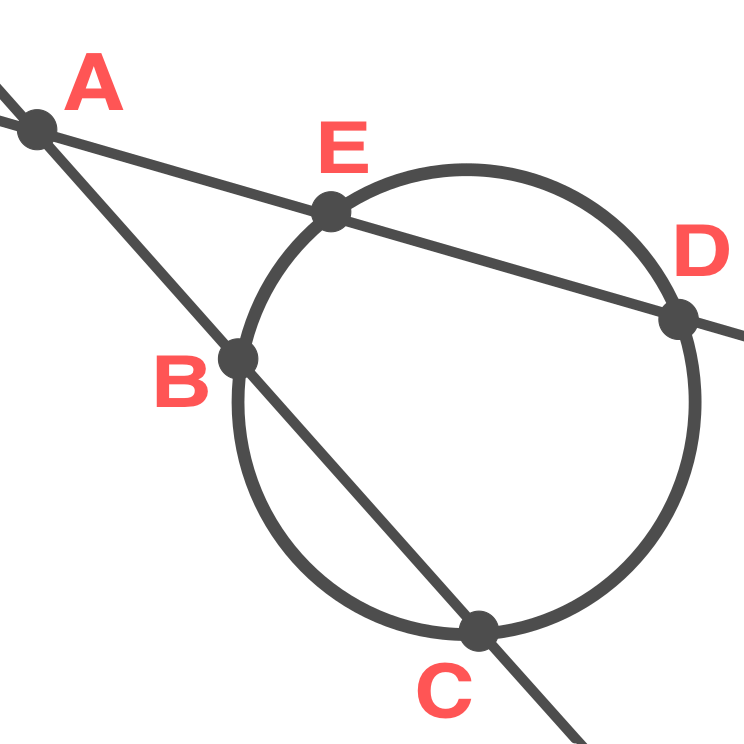
\angle CAE = \frac{1}{2} (CE-BD)

Secant and Tangent

x = \frac{1}{2} (BD-BC)